Richards' equation

The use of Richards’ equation allows conditions being simulated where the assumptions contained in the cascading model do not hold, for instance when the water level rises (due to a permanent or temporary water table), and when the soil water content is high, or when relevant texture differences are present among soil horizons. Richards’ equation is based on physical concept. The model assumes that water flow between two points is a function of the pressure gradient between the points and of the hydraulic conductivity. Consequently, water retention curves and hydraulic conductivity are needed as a function of soil water content and/or water pressure. In the last decades, with the development of computers, several numerical routines have been developed to solve Richards’ equation (Haverkamp, 1977; Ross, 1990; Pan and Wierenga, 1995; van Dam and Feddes, 2000). The difficulty in solving the Richards’ equation is due to the fact that it is a parabolic differential equation and to the non-linearity of hydraulic functions that correlates water content, water potential and hydraulic conductivity. The large changes in soil water contents at the proximity of soil surface (due to evaporation and precipitation) further complicates the solution introducing problems of numerical instability. The calculated water flows depend on the structure of the numerical outline, the interval time and the steps spaces (van Genuchten, 1982; Milly, 1985; Celia et al., 1990; Miller et al., 1998). Here the approach of van Dam and Feddes (2000) will be adopted, because it allows for the presence of water table in the soil profile. This method of solving Richard’s equation has already undergone extensive testing because it is implemented in the SWAP model (van Dam et al., 1997).

Combination of Darcy’s law and the principle of mass conservation, results in Richard’s equation which, in the vertical dimension (1D), can be written as:

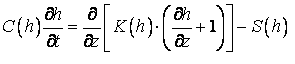
where C is the differential water capacity (dθ/dh) (l-1), θ is the water content (l3 l-3), h is the soil water pressure head (L), t is the time (t), K is the unsaturated hydraulic conductivity (l t-1), z is the vertical coordinate (positive upward) (L), and S the root water extraction (l-3 l-3 t-1).

Created with the Personal Edition of HelpNDoc: Produce Kindle eBooks easily